Home >> Quantum, wave-particle duality

The De Broglie Equation

In 1924 Louis de Broglie proposed that matter had a wave nature. His famous equation links the momentum 'p' of a particle with its wavelength ' λ' (the De Broglie wavelength).

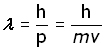
Note, these are not matter waves. The amplitude of the wave at a point represents the probability of the particle's position.
The relation was verified by Davisson and Germer in 1927 by electron diffraction in crystals.

...more on this in the section 'electron diffraction'

Optical interference from a double slit

This was first demonstrated by Thomas Young in 1801.

Two light sources can produce interference provided:

1. the sources are coherent*

2. the wave amplitude is approx. the same

* a constant phase difference must exist between each wave (so the frequency must be the same).

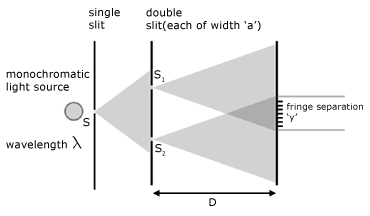
Young's double-slit equation is:

λ  wavelength
y   fringe separation adjacent bright/dark fringes
a   slit separation
D   perpendicular distance between slit plane and screen

experimental observations

1. fringe separation increases by increasing separation 'D'

2. fringe separation decreases by decreasing 'a'

3. increasing the slit width 'a' increases fringe brightness but
    blurring occurs

4. moving the single slit (S₁) closer to the double slit (S₁ S₂)
    increases fringe brightness with no effect on separation

back to top

Quantum effects

Quantum mechanics explains the fringe pattern from particle path probabilities.

The position of particles is described mathematically by probability waves. As in the classical case, amplitudes add and subtract from one another.  An interference pattern is the result.

Considering photons as particles, if we reduce the emission rate, photons go through the slits one at a time*, and after enough time has elapsed, an interference pattern is built up.

*Strictly speaking, according to quantum mechanics, a photon can go through BOTH slits at the same time.

Similar interference patterns are obtained by firing particles(eg electrons) at a double slit. The bottom line is that waves behave as particles and vice versa.

back to top

Wavelengths compared

X-rays waves have a wavelength of the order of 10^-11 m travelling at the speed of light at approximately 3 x 10⁸ ms^-1.

We can get an idea of wavelength for particles, but it must be remembered that their wavelength depends on velocity, which is variable.

So as an example let's take an electron travelling at 10% the speed of light.

m_e = 9.1×10^-31     v = 3 x 10⁷ ms^-1      h = 6.6×10^-34 J.s

using the De Broglie equation,

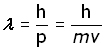
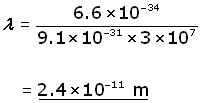
The electron wavelength travelling at 10% the speed of light has approximately the wavelength of X-rays. Since wavelength and velocity are inversely proportional, the higher the velocity, the shorter the wavelength. Thus shorter wavelengths, approaching that of gamma rays, can be obtained with higher electron velocities.

Using more massive particles, for example neutrons, produces even shorter wavelengths. Neutrons are approximately 2000 times the mass of electrons. So their wavelength for the same velocity, will be 1/2000 th(approx 1.4 x 10^-14 m).

There is another wavelength pertaining to particles that is different from the De Broglie wavelength. This is called the Compton wavelength.

The Compton wavelength has particular relevance in quantum mechanics. It was introduced by Arthur Compton as part of his theory on the scattering of photons by electrons(Compton scattering).

By definition, the Compton wavelength of a particle is the wavelength of a photon with the same energy as the rest-mass energy of the particle.

back to top

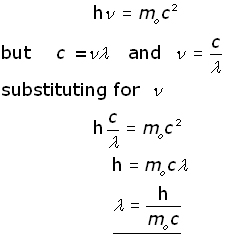
[ About ] [ FAQ ] [ Links ] [ Terms & Conditions ] [ Privacy ] [ Site Map ] [ Contact ]